


The Editors of Encyclopaedia Britannica (2019). Albert Einstein and the most elemental atomic theory - ANS / Newswire. But until 1932 everyone believed the atom had the nucleus which was positively charged and the electrons negatively charged.īowersox, P. His model is done by using quantum mechanics, and the model's name is referred to as the quantum mechanical model of the atom. They don't really have a color, we just use that idea because it's sort of helpful, just like using the idea of positive and negative for charges is helpful.Schrodinger enhances the atomic model, so it can show the electrons orbits in 3D on a 2D plane. IN the field of quantum chromodynamics, we say that particles have a color. Without the neutrally charged neutrons, the protons would mutually repel each other, and the nucleus would fall apart. It's just the name that we've given a quark that has certain properties. Chemistry 3D Models 0 Bohr's model of Carbon Bohr model of the atom According to Bohr (1913), electrons moved in distinctive energy levels or orbitals around the positively charged nucleus of one proton. There's nothing unusually strange about the strange quark. There's a charmed quark and a strange quark. THere's nothing "up-ish" about an up quark. "Up" and "down" don't have any meaning other than to identify the type of quark.
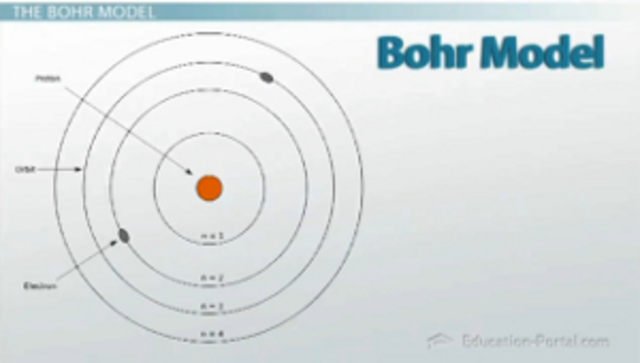
A quark can be an up quark or a down quark. We have other properties like this for other particles. It's just a characteristic that particles have or don't have, and we know what the effect is of having or not having that property. "What is spin" doesn't have any deeper answer than does the question "what is charge". But there is no point in trying to answer "what is electron spin" by referring to some familiar object like a ball or a planet, because electrons are not balls or planets, they are their own thing, and you just have to accept that they have a property whose effects we understand very well, and we happen to have named it spin. Still, they have a property that works sort of like an orbit (orbitals) and they have a property that works sort of like rotation about an axis (spin). Using this concept actually helped a bit, just like Bohr's imagination of little orbits helped a bit, but again, the electrons are not like little planets: they don't revolve around the nucleus, and they don't rotate on their axes. Just as Bohr imagined that the atoms were little planets revolving around the nucleus as though it were a sun, other scientists tried to extend that idea by imagining that the little planets, just like real planets, had spin. It got named spin back when people were working with the Bohr model and trying to extend it to atoms beyond hydrogen. Spin is just a property that electrons (and other particles) have. For another layer, you can take the fact that you can never cool anything down to exactly 0K (-273.15C) (although you can get close) and so nothing will ever have 0 velocity. (1 value in a range of reals is like trying to throw a dart at a dartboard with an infinitely thin wire and hitting the wire). The Bohr model of the atom, a radical departure from earlier, classical descriptions, was the first that incorporated quantum theory and was the predecessor of wholly quantum-mechanical models. With this uncertainty, the velocity is almost definitely not 0. Bohr model, description of the structure of atoms, especially that of hydrogen, proposed (1913) by the Danish physicist Niels Bohr. As we can't physically measure to perfect accuracy, there is an uncertainty in both measurements of the degree that we know it's probably stationary and it's probably 'over there'. So, if you know with 0 uncertainty what the velocity is, then you have no idea where it is, and all future involvement of the particle is pretty much irrelevant (how is the electron going to diffract around an atom if the electron is in a different galaxy?). The square (probability function) shows that it has an equal chance of being anywhere. If you take the infinite wavelength interpretation, then it would be nearly 0 (1/inf) but constant everywhere. Isn't to do with the fact that the velocity is not quite 0? if you know it is exactly 0 then the uncertainty in the position is infinite as well (momentum is a function of velocity, so delta P = 0 -> delta V = 0 -> delta X = inf) therefore it has an equal probability of being anywhere.


 0 kommentar(er)
0 kommentar(er)
